Research: Slanted QGMy thesis work consists of two parts: A slanted quasi-geostrophic (QG) model used to study geophysical turbulence; and a protoplanetary disk model of the gas that surrounds young stars.The quasi-geostrophic (QG) potential vorticity equation is a reduced model for stratified, rotating flows which is often employed in atmospheric and ocean dynamics. QG, first derived by Charney in 1948, has been successful in analytic models of Rossby waves, cyclogenesis, and ocean basin circulation, as well as fundamental numerical models. A weakness of QG is that the Rossby number must be small (i.e. rotation is stronger than advection), so it is only applicable at mid- and high-latitudes. I am working on an alternate asymptotic derivation of the primitive equations dubbed "Slanted QG" where the full rotation vector is kept, not just the vertical component of rotation as in standard QG. This may be an appropriate model to study the dynamics of stratified flows near the equator. I have created a 3D periodic pseudo-spectral numerical model of slanted QG to study vortex behavior and the energy cascade in this regime. 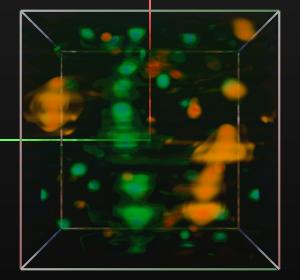
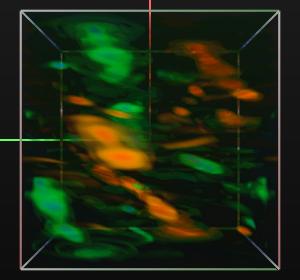
These images show three dimensional volume rendering of vorticity produced at the end of a run-down simulation using my model. Orange is positive vorticity and green is negative vorticity. Like-signed vortices merge within each horizontal level and align on neighboring levels. Here vortex columns align with the axis of rotation which is vertical in standard QG (left) and tilted at 45 degrees in a slanted QG simulation (right). The figures below show standard statistics collected in turbulence simulations, like the spectrum, enstrophy, and vorticity distribution. 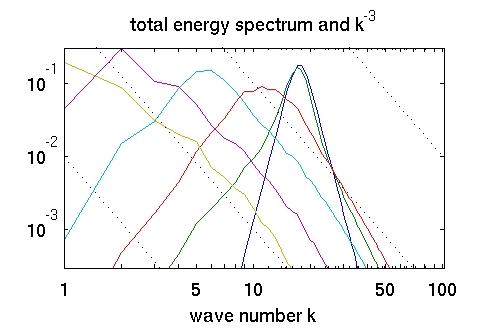

ComputingSee the Applied Math Department's Itanium Lab web page. |

